This page is intended to be a look at some of the key technical aspects of the B3 Hammond organ that contribute to the “Hammond sound”. My personal slant is towards copying this sound using other technology. Perhaps one day I’ll build a “clonewheel” organ, but then again, Clavia might have nailed it! Still, building an organ would be a nice project.
Contents
- Drawbars
- Foldback
- Key Click
- Harmonic Leakage
- Chorus/Vibrato scanner
- The problems with copying the B3
- The easy parts of the B3
- Working out the frequencies of the tones used
- What frequencies are used?
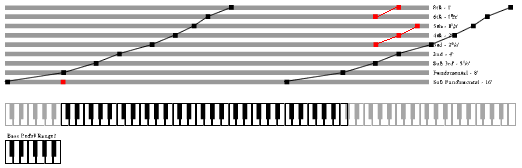
Drawbars
The harmonics, footages, and notes used on the Hammond drawbars are shown below:
As can be seen, for each note, the harmonics go three octaves above it, and one octave below.
The basic pitch of the instrument runs from tone 13 to tone 73. This pitch is provided by the 3rd drawbar, the fundamental.
The 5⅓’ footage is oddly placed as the second drawbar, rather than in its logical position between the fundamental and 2nd harmonic, presumably because it is labelled as the ‘sub-3rd harmonic’.
Foldback
Harmonic foldback is used to reduce the required number of pitches at both ends of the keyboard. It affects the 3rd and subsequent harmonics at the top, and the sub-fundamental harmonic at the bottom. This is shown in the following table, which shows the tonewheels (1-91) used for each harmonic
| Lower Foldback | Top Foldback | |||
|---|---|---|---|---|
| Sub-Fundamental | 13-24 | 13-61 | ||
| Sub-Third | 20-80 | |||
| Fundamental | 13-73 | |||
| Second | 25-85 | |||
| Third | 31-91 | 80 | ||
| Fourth | 37-91 | 80-85 | ||
| Fifth | 41-91 | 80-89 | ||
| Sixth | 44-91 | 80-91 | 80 | |
| Eighth | 49-91 | 80-91 | 80-85 | |
The 3rd harmonic repeats the highest C. The 4th harmonic repeats from tone 56, G of the top octave. The 5th harmonic repeats from tone 52, D# of the top octave. The 7th harmonic repeats from tone 49, the lower C of the top octave. The 8th harmonic is repeated from tone 44, G in the fourth octave. Note that the 6th and 8th harmonics actually repeat some tones twice.
Since the lower tones that are folded back are actually present in the instrument for the pedals, it is possible to rewire a Hammond for true bass, with no lower foldback. The quirk is that the lowest octave of tonewheels are cut with a more complex shape and provide a waveform that is closer to a squarewave, with some 3rd and 5th harmonics present.
The diagram below shows the effect of the harmonic foldback on the top and bottom notes of the keyboard. The lowest note has its sub-fundamental folded back, even though the tone generator goes low enough, represented by the width of the grey bars. At the top, there are five tones which ‘go off the top’ of the generator and are folded back onto the top octave provided. Notice that this means that if you play the top C, it actually includes two of the same tones (tones 80 and 85) three times over!
In theory the full 5 octaves of the B3 keyboard would require 61 basic tones, plus 3 octaves above for harmonics and one octave below for the sub-fundamental harmonic. This gives a total of nine octaves, or 61+36+12 = 109 tones. Since there are only 91 tones available, the need for foldback is clear.
Key Click
The mechanical key contacts on the B3 have audio level signals present on them. Switching these directly through to the output caused audible clicks. These clicks have become a signature part of the Hammond sound. The clicking is caused by a combination of the nine key contacts not shutting simultaneously, and contact bounce exacerbated by dirty contacts. This causes a random rapid switching of the signal in the initial portion of the note. Since this switching introduces transients, we hear this as a sound with much random high frequency content – a click. This could be simulated either by adding in transient noise, or by electronically simulating contact bounce.
Harmonic Leakage
The Hammond tonewheel generator contains a series of metal dividers, which break the generator up into “bins”. Each bin contains 2 tonewheels which are connected to the same driven gear. There is a certain amount of magnetic leakage between tones that are in the same bin, so it is possible to hear harmonic leakage either four octaves above or below the required tone. Good copies of the Hammond include this leakage.
Chorus/Vibrato scanner
The Hammond chorus/vibrato circuit has become famous in its own right, with organs that have a “real” scanner-based vibrato being more valuable than those without. So what is this circuit and how does it work?
In short, it is a 9 stage delay line. The delay is produced using LC phase shift circuits. It can produce only a very short delay of around 1mS . A variable delay is required for vibrato or chorus, and this is generated by the rotating scanner arm picking up signals from each stage of the delay in turn. Because of the way this is done (air-gap capacitor, essentially) the effect is a fade between one stage and the next. The scanner is set to scan each tap from 1 through to 9 and then back again 9 to 1. This is equivalent to a triangle wave modulation of the delay time. The complete cycle, from 1 to 9 and back, is 16 steps. Although three depth settings are available for each of the two effects (VIB1, VIB2, VIB3, CHORUS1, CHORUS2, CHORUS3) on the B3, there is no control of rate, which is set at a constant 7Hz. Any good copy has to be able to provide this speed.
Juergen Haible has spent some time copying the original Hammond scanner circuit and producing a modern copy. Unfortunately his circuit is not currently available online. It uses a real LC phase-shift delay line, coupled to a pair of VCAs and other circuitry to do the fading from one stage to the next. This apparently produces an extremely close copy of the original sound, without any moving parts.
Other, less stringent, copies would be possible using BBD devices. The problem with these would be getting the extremely short delay times required. Most chips would have too many stages and too slow a clock to get the times required. These also provide the same delay to all frequencies, not something which is a feature of Hammond’s LC phase-shift delay.
Perhaps a more promising route would be to use 9 op-amp based phase shifters and then scan between the outputs of these using a circuit much like Juergen’s.
Problems with copying the B3
There are various problems with copying the B3. They are:
Generating 91 individual sine wave tones
This is not so easy to do, and even harder at reasonable cost. Ideally, the waves should not have fixed phase relationships between related notes, although they do have a reasonably fixed relationship in the original organ. The spring couplings between stages in the tone generator give this some fluidity though. This fixed-but-not-fixed phase relationship is another interesting aspect of the technology, but one with an unknown influence on the sound.
Switching the tones to the busbars
The original organs have nine contacts under each key. Even this is insufficient, since they rob one contact from a harmonic when the percussion is switched on. For every key that is pressed down, (at least) nine individual frequencies must be passed to nine separate output mixers, and a signal must be passed to a percussion circuit. This makes a total of 61×10 contacts required for each of two manuals (ignoring the pedals!) for a grand total of 1220 switch contacts, although as mentioned Hammond reduced this a little.
If there is a way to to wire this up without it looking like the web of a spider suffering obsessive-compulsive disorder, I haven’t seen it. Even multiplexing doesn’t seem to help, since it simply moves the problem back to become a question of getting the correct tones to the multiplexers.
This is the fundamental problem with any fully polyphonic organ – and all the best ones are.
The easy parts of the B3
The output circuits are pretty straightforward. The busbars are simple mixer circuits that sum all the notes pressed down, and the drawbars control the output volume from each harmonic’s mixer. These harmonics are then mixed in the final output mixer before being amplified.
Getting this to sound like a B3 is a question of tone and technology – using tubes not transistors and so forth. If everything else is ok in your clonewheel organ, and you plug it into a valve amp Leslie speaker, it’ll sound like a Hammond.
Working out the frequencies of the tones used
The frequency of a particular tone depends on a number of things:
- M – The main motor speed, 20 revs/second (1200 rpm)
- T – The number of teeth on the tonewheel
- R – The gear ratio. This is equal to the number of teeth on the driving gear / number of teeth on driven gear.
The ratios in the Hammond are as follows:
| Note | Driving (A) | Driven (B) | Ratio (A/B) |
|---|---|---|---|
| C | 85 | 104 | 0.817307692 |
| C# | 71 | 82 | 0.865853659 |
| D | 67 | 73 | 0.917808219 |
| D# | 105 | 108 | 0.972222222 |
| E | 103 | 100 | 1.030000000 |
| F | 84 | 77 | 1.090909091 |
| F# | 74 | 64 | 1.156250000 |
| G | 98 | 80 | 1.225000000 |
| G# | 96 | 74 | 1.297297297 |
| A | 88 | 64 | 1.375000000 |
| A# | 67 | 46 | 1.456521739 |
| B | 108 | 70 | 1.542857143 |
The formula for the exact frequency of a tone is:
Frequency F (Hz) = M * T * R
Thus for Middle A:
20 * 16 * (88 / 64) = 440Hz
The As are the only notes on the Hammond which agree exactly with the equal tempered scale. The notes which are the farthest off pitch in the first seven octaves are the G#s. They are 0.69 cents flat from the correct pitch.
For C# = 4434Hz: 20 * 192 * (74/64) = 4440Hz
Essentially, the gear ratios determine the note, whilst the number of teeth on the tone wheel determines the octave. Hence all the tones in a single octave use wheels with the same number of teeth. The 91 frequency tone generator uses:
- 12 tonewheels of 2 teeth
- 12 tonewheels of 4 teeth
- 12 tonewheels of 8 teeth
- 12 tonewheels of 16 teeth
- 12 tonewheels of 32 teeth
- 12 tonewheels of 64 teeth
- 12 tonewheels of 128 teeth
- 7 tonewheels of 192 teeth
The last (top) octave is unusual, since Hammond could not cut wheels with 256 teeth. Instead they used wheels with 192 teeth and used the gear ratios from the F below upwards. This means the top half octave is farther off pitch due to the number of teeth on the tonewheel not equaling 256. In the top half octave, the C# is the farthest off pitch, about 1.93 cents sharp. This is still less than the 6 cents that supposed to be the minimum discernible pitch difference.
Equal temprament tuning means that it is impossible to find exact harmonics on the keyboard – each tone is a “best fit” rather than the exact value. However, the Hammond doesn’t use a true equal temperament tuning, but rather its own approximation. In the case of the 6th, 3rd and sub-3rd harmonics, this leads to them being slightly closer to the true value than equal temperament, and in the case of the 5th, slightly worse. The differences are present, but very slight and probably not significant. Ignoring the top half-octave which is rather different, the results are summarized in the table below:
| Harmonic | True harmonic ratio | Equal temperament ratio | Hammond ratio |
|---|---|---|---|
| Sub-fundamental | 0.5 | 0.5 | 0.5 |
| Fundamental | 1 | 1 | 1 |
| Sub 3rd | 1.5 | 1.498307077 | 1.498823530 |
| 2nd | 2 | 2 | 2 |
| 3rd | 3 | 2.996614154 | 2.997647060 |
| 4th | 4 | 4 | 4 |
| 5th | 5 | 5.039684200 | 5.040941178 |
| 6th | 6 | 5.993228307 | 5.995294120 |
| 8th | 8 | 8 | 8 |
What frequencies are used?
The bottom octave runs from 32.69Hz to 65.38Hz. These tones are the complex tonewheels and are only available on the pedals.
The tones for the manuals run from 65.38Hz (the lowest C) to 2092.31Hz (the highest C). The tone generator also generates harmonics another octave and a bit above this, to the C above (4184.62Hz) and finally to the F# above that (5924.62Hz). This is the highest pitch in the organ.

Hi there,
Interesting article. I have been trying to match the information given to help with on problem on my Hammond SK2 digital clone. One note at the top of the keyboard (5F) seems much louder than others in the same area. I can soften this by pushing in the second drawbar to about 6 but I’d rather not, given that 88800000 is a common B3 setting for jazz. The SK2 has 96 digital tonewheels with editable parameters. Do you happen to know which tonewheel (or wheels) corresponds to this high F note? Then maybe I can adjust the parameters to soften the sound.
Many thanks
Jeff Wells
I don’t know exactly, but I think you might be able to find out over at HammondWiki.
Tom
Hi Tom,
I’ve created a monophonic synthesiser using an Arduino and a look-up table.
The question is, how to change the table to create a”classic” Hammond sound? (albeit without chorus / tremolo / Leslie effects).
What I need is an oscillograph of the waveform from a working organ, set up to mimic one of the “greats” such as A Whiter Shade Of Pale (one of my favourites). 30-seconds of middle “C” would also be perfect. I’m told that 888000000 is “the” setting I should go for. I can then alter the amplitude values in the wavetable to suite. Voila!
Any ideas?
Jim
Hi Jim,
Yes, I can help a bit, but I don’t think you’ll like the answers! While it is possible (simple, even) to generate a waveform with the correct amounts of the required harmonics using a fixed wavetable, it won’t sound like a Hammond. Instead, it’ll sound more like a TOG-based organs from the Seventies, although it isn’t identical to those either. The difference is that although the Hammond harmonics are close to exactl harmonics ratios, they’re not spot on. This means that two consecutive cycles of a mixed Hammond waveform are not the same. Think about it: If you add sine waves at 100Hz and 200Hz, you get a shape that will be the same for each cycle. If you add sine waves at 99Hz and 201Hz, you’ll get a wave shape that changes over time. That’s the Hammond situation, and (to a lesser extent) the situation with TOG-based organs.
That said, a fixed wavetable which has equal amounts of the 1st, 2nd, 4th, and 8th Harmonics has a very identifiably “electric organ” sound. Try it. It might be close enough for rock’n’roll. If you want to really get closer, you need to look more at the (exact) frequencies the Hammond generated.
Your idea of sampling 30 seconds of middle C is probably going to get you closer, but looping such a sample might not be easy, and 30 seconds is quite a lot of memory. And if it works, you’ve built a sampler, not an organ!
HTH,
Tom
Hi Tom,
Thanks very much for your reply.
I also looked into how the Hammond produces it sound, and found as you said that the waveforms and tones produced are, for instance, not exactly equal temperament, I suppose because it would be difficult or impossible to cut tonewheels all running at the same speed to create the exact fequencies required. Also, being a mechanical system, there will be backlash and mechanical vibration etc that will muddify thing a little bit.
Anyway, I finally found a real (1960’s, with tubes) Hammond, and in reality, the notes are a bit “boring” being more or less sinewaves, and the instrument only became interesting when I tricked around with the 9 drawbars. I recorded a few seconds of Middle “C” with my phone in the shop (with permission!) and dumped the .wav file into my PC using “Reaper”. I then used Reaper’s graphics capability to isolate a typical single cycle, and by a long & tedious process, printed the waveshape onto “graph paper” which I custom-made with 256 divisions in the X & Y directions.
I then read the amplitude for each interval (1/256th of the waveform) & type the values into an Excel spreadsheet, (a) to check the values using Excel’s chart facility and (b) to allow me to add in commas etc to paste intoa wavetable.
I put the wavetable into the Arduino, and you know, it doesn’t sound bad. I have used Reaper in the past to create the 7Hz Hammond tremolo & vibrato effects, and also to (sort of) mimic the phase effects that you get with a Leslie speaker, and the combination with the Arduino synth suits the sort of 60’s tracks I like to play around with.
I dare say I should create another 9 or 10 wavetables on the grounds that the Hammond has different waveforms up and down the keyboard for the same drawbar settings, but I might as well buy the Hammond instead & save a lot of time!
Wow Jim! Nice work! There’s nothing like the “experimental approach” to really find out whether an idea is a goer or not.
Original Hammonds aren’t your only option these days, either – the digital clones are really that good now. Even Hammond make one!
Tom
Jim McLay: The reason why the tonewheel Hammond can only approximate equal-temperament (very well, I will add) is because the frequencies are the result of gear ratios between the numbers of actual physical teeth on the tonewheels. An equal division of the octave is a process of taking roots of the 2:1 frequency ratio which defines the octave, thus they are irrational divisions, not ratios. The standard 12-tone equal-temperament defines the semitone as the 12th root of 2. Obviously, the number of actual physical teeth must necessarily be integers, so a relationship between two of them will be a ratio with integer terms. Hammond cleverly designed a set of ratios which does an excellent job of approximating 12-tone equal-temperament. Tuning theorists call this an RI (“Rational Intonation”), which includes JI (“Just Intonation”) as subsets, the difference being that listeners are not expected to perceive the intervals as ratios in RI. And note also that the harmonics on the drawbars will also have the same RI frequencies. The 16′, 8′, 4′, 2′, and 1′ drawbars are in exact power-of-2 (octaves) ratios. The 5 1/3′, 2 2/3′, and 1 1/3′ drawbars are slightly off from true 3:2, 3:1, and 6:1 ratios respectively, but are very close, less than 1 cent. The only drawbar which is really different is the 1 3/5′, giving a frequency ~14 cents higher than the true 5:1 ratio of the pipe organ stops it was originally intended to emulate.
http://tonalsoft.com/enc/h/hammond-organ.aspx
Thanks Joe. I hadn’t seen your site before – that’s really great.
Hello Tom,
I know this reply is more than 3 years overdue, but maybe still of interest. Try using 2 identical wave-tables. The sampling routine should be implemented such that the frequencies of the signals generated from the 2 oscillators are slightly different, i.e. the output frequency of one oscillator is “detuned” by a few cents relative to the other. This technique is used in pipe organs and is known as “voix celeste” (heavenly voice). This won’t get you a whole lot closer to an authentic Hammond sound, but it will produce a much richer and more musically interesting sound than a single wave-table oscillator.
Cheers – Mike [www.mjbauer.biz]
Hi, great info.
I,m making a Hammond clone in DSPIC,
i,m curous about this : is each note amplified ?, or does it add up to a maximum sound level per tonewheel ?
thanks
I’m not completely sure. I’ve read various things saying that adding notes on a Hammond gives a “compressed” type of sound because of the way the extra tones are added (so for example two tones aren’t x2 louder, but only x1.75 or something) but I don’t know if this is accurate, and I’ve never studied the schematics of the busbars and drawbars to discover for myself. Sorry!
Tom
I think you may find some useful info in the original patent:
https://docs.google.com/viewer?url=patentimages.storage.googleapis.com/pdfs/US1956350.pdf
page 13 (that is page 31 of the pdf ) in the “Elimination of ‘robbing'” section
Hi, one of many useful and informative articles about the Hammond Organ, thank you.
I’ve embarked on a bit of a folly… to design and build a pure analogue organ based on Hammond’s principles but using modern(ish) tech. Using analogue IC’s yes, but not a clock or a square wave in sight!
Full piano range, A0 to C8. with around 135 individual pure analogue tone generators, A1 (13.75 Hz) to C11 (33.488 kHz) though I’ll probably ditch the upper ones as being too high to make any difference. Each with around a +/- 3% tuneable range and frequency and amplitude stable(ish).
88 (one for each key) 9 input voltage controlled mixers for mixing the fundamental, sub, and other 7 harmonics. The 9 ‘draw-bars’ set the control voltage for each of the 9 channels of the 88 mixers with inputs to the 9 mixer channels from the relevant 9 tone generators for that particular key. The output from each mixer is gated by the keyboard.
Finally a simple 88 input mixer for mixing the outputs from each of the key’s mixers, providing true 88 key polyphony.
Thereafter, reverb, vibrato, keyboard click, Leslie effect could be added. I have an idea for adding a VCA to the output of the key mixers controlled by how hard/fast a key is hit to control the loudness, but that’d not for now.
The electronics are designed. Next it’s on with the build as time and finance (800 voltage controlled mixer chips (probably Coolaudio V2181’s) do not come cheaply) permits starting with a single octave, 12 keys, 12 9-input voltage controlled mixers and 60 (ish) tone generators. Just to see how it sounds and if’s worth pursuing.
I could simulate it but methinks all that wiring, analogue electronics will bring it’s own characteristics to the final sound.
It sounds like an amazing project! Good luck!
One thought: Instead of 88 9-input mixers, you should have 9 88-input mixers. Then your drawbars can mix the outputs of those mixers down to the final output – that saves you a lot of VCAs and complexity. Not that you won’t still have plenty!
Tom
Sounds amazing. How is the work going? 🙂
It’s important, when adding the contributions of different keys to the volume of the same tone wheel, NOT to add them linearly. When uncorrelated sounds are added, they add in the power domain, not the voltage domain, so adding two different 1V signals gives you 1.414V, not 2V. If you add two identical signals, then 1V + 1V = 2V, which gives you four times the power, which sounds all wrong.
While working for Rodgers Instruments back in the 1990s, I did a good Hammond simulation in their W-5000, using Roland XP chips for the actual wavetable readout. I took all the contributions from the individual notes, squared them, added them up, then took the square root, for a true RMS sum. That was a pretty good match for what a Hammond sounds like. I don’t know, however, how a real Hammond approximates an RMS sum, but I suspect it’s only an approximation.
A very interesting article, but a very important subject is not mentioned. Manuel-Tapering. The difference in wire-resistance is the main reason for the „screaming“ Hammond B/A-Series and a very important difference to the M-Series-Sound. See: http://www.dairiki.org/HammondWiki/ManualTapering
“The quirk is that the lowest octave of tonewheels are cut with a more complex shape and provide a waveform that is closer to a squarewave, with some 3rd and 5th harmonics present.”
This may have been an intentional imitation of a pipe organ; many small/medium church organs use stopped (Gedeckt) pipes in the pedal ranks, so the waveform is sort of squarish.
Very interesting, David! Thanks, I didn’t know that.
Hi, I have a Hammond Elegante, and have a problem with the Bb & A pedals. I removed the pedalboard only to find the white buttons for those pedals have fallen into the bottom of the organ. Is there a way to get them out to re-connect that section of the pedalboard?
Obviously Lauren’s Hammond was a absolute genius as he did not even play music.
And they say he could not sing on key ether.
I had no idea about the different gear ratios and the teeth on the tone wheel’s.
I only knew that there were 91 tone wheels.
Still this 1930s technology is still absolutely amazing today still. I own an A100 and Leslie.
Hi,
Great article, thanks!
As regards “The last (top) octave is unusual, since Hammond could not cut wheels with 256 teeth. Instead they used wheels with 192 teeth and used the gear ratios from the F below upwards.” and “The tones for the manuals run from 65.38Hz (the lowest C) to 2092.31Hz (the highest C). The tone generator also generates harmonics another octave and a bit above this, to the C above (4184.62Hz) and finally to the F# above that (5924.62Hz). This is the highest pitch in the organ.” …. when I put the values into Excel I get slightly different frequencies from you for the high end. For example:
Note Tone Teeth Driving Driven Ratio Generated Hz
C8 85 192 84 77 1.090909091 4189.091 (vs your 4184.62)
F8# 91 192 108 70 1.542857143 5924.571 (vs your 5924.62)
Hello gentlemen.
Excellent article.
I have HAMMOND model B preamplifier (possible with serial number S4294) and looking for technical help. Can anyone tell me how big the output voltage (Vrms, Vpp, dBV, etc) is from the matching transformer, or what is the input signal range of preamplifier.
Thank you in advance.
Sincerely,
Vitaly.
If anyone reading this can help Vitaly out, please leave a comment. Thanks.
Thank you Tom for assistance.
Sincerely,
Vitaly
Great article. It has inspired me to attempt to replicate as many aspects of the B3 as I can with my DX7iiFD. I think the DX7iiFD is well suited for this and with a bit of thoughtful programming, can probably approximate most of the items mentioned in this article (including foldback!). If anyone else has interest in my experiment, or would like to collaborate with me, please reach out to me.
Interesting idea! In some ways, the DX7 couldn’t be *further* from the Hammond organ, but then in other ways, it’s actually quite close – both instruments are *fundamentally* based on sine waves, for example.
Good luck!